Estimation of seepage losses using empirical formulas in comparisons with field test from Hajira Branch Canal

Khyati V. Mistry^{* *}Correspondence: Khyati V. Mistry, Department of Civil Engineering, C.G. Patel Institute of Technology, Bardoli-394350, Gujarat, India, Email:

Author info »

Introduction

Canals are considered as one of the significant conveyance system of any irrigation project [1]. A canal may be of rigid boundary or mobile boundary canal [2]. Compare to the lined or rigid boundary canal, an unlined canal is subjected to a significant water loss due to the seepage [2]. Seepage can be defined as the movement of the water in or out from the voids present in the bed and sides of the earthen unlined canal [3]. Due to the seepage, more than 50% of usable water is get lost [1]. Seepage loss also causes depletion in freshwater resources, water logging, salinization, and ground water contamination [2]. Therefore, it is essential to determine seepage losses to reduce the amount of water loss and to serve the maximum command area. Most of the canals are earthen canal which is inexpensive to construct but it loses 50 percent or more water due to seepage. These type of canals are more liable to get eroded due to weed growth and other problems which gently reducing the effectiveness of over the time. In old earthen irrigation canals in considerable water loss is take place due to seepage from its sides & bed which results into low conveyance efficiency of canal. Earthen canals are also get clogged due to weed growth which reduces its water carrying capacity. In the present study, Hazira branch canal is taken in the consideration. Seepage losses are determined by indirect method (Particle size distribution), and also by empirical formulas.

Need of study

1. Seepage from irrigation canals results in waterlogging of valuable agricultural land and also makes land excessive alkaline. So, it constitutes a serious agricultural problem.

2. Insufficient maintenance, problem of leakages and seepages is also present in command area resulting in wastage of water, water logging etc.

3. Seepage rates in earthen canals are very high as 45%, so if these rates are reduced considerable amount of water is save which can be used for irrigating more area.

Materials and Methods

Study area

Hajira is known as the industrial hub of the India. It is located in Surat, Gujarat at coordinates of 21.13451°N 72.64772°E. It is 8 kms away from the Arabian Sea. It is having the total area of 168 kms. It is base for major industries and shipping facilities like Essar, Kribhco, Shell, Larsen & Toubro, NTPC, ONGC, GAIL, Gujarat State Petroleum Corporation, UltraTech Cement and Reliance Industries. Hajira Branch Canal was constructed as unlined canals in 1954 and 1975 respectively. Hajira branch canal is taking off from kakarapar right bank main canal having total discharge of 1207 cusecs and having total length of 34 km (Figures 1 and 2 and Table 1).

Table 1: General details (Dimensions and characteristics of different sections).

Figure 1: Index map.

Figure 2: Methodology outline.

Estimation of seepage losses

Seepage from an irrigation canal is usually measured by following methods.

1) Direct measurement method

2) Indirect measurement method

Direct measurement method

Seepage from an irrigation canal is usually measured by direct measurement methods:

I. Ponding test.

II. Inflow-outflow method

III. Seepage meter method

IV. Constant and variable head parameters

Direct method cannot be used as prevailing site condition and does not permit the same and hence, it is decided that the indirect method of finding the seepage by conducting the seepage analysis of the soil in the laboratory was performed.

Indirect measurement method

Grain size analysis (Sieve analysis): The soil samples are taken for grain size distribution by mechanical analysis in the laboratory. The samples are oven dried first then perform experiment and find out the percentage finer of the soil and distribute the soil by grade sizes by Wentworth scale.

Sieve analysis

1. Write down the weight of each sieve as well as the bottom pan to be used in the analysis.

2. Record the weight of the given dry soil sample.

3. Make sure that all the sieves are clean, and assemble them in the ascending order of sieve numbers (4.75 mm sieve at top and 75-micron sieve at bottom).

4. Place the pan below 75-micron sieve. Carefully pour the soil sample into the top sieve and place the cap over it.

5. Place the sieve stack in the mechanical shaker and shake for 10 minutes.

6. Remove the stack from the shaker and carefully weigh and record the weight of each sieve with its retained soil. In addition, remember to weigh and record the weight of the bottom pan with its retained fine soil.

Estimation from grain size

Allen Hazen derived an empirical formula for approximating hydraulic conductivity from grain size analysis:

K = C(D₁₀)²

Where,

Hazen's empirical coefficient, which takes a value between 0.0 and 1.5 with an average value of 1.0. A.F. Salarashayeri and M. Siosemarde give C as usually taken between 1.0 and 1.5, with D in mm and K in cm/s.

D₁₀ is the diameter of the 10 percentile grain size of the material.

By using earthen dam seepage line concept seepage rate is found by using Q=KS.

General values of permeability for different soils are given in Table 2.

Table 2: General values of permeability.

Results and Discussion

In the present study, a total of 20 sections of the Hajira branch canal are taken into consideration, starting from Rd. 15.6 to Rd. 87.554. From all the sections, soil samples were collected, and sieve analysis has been performed. The field test results of the seepage loss from the Hajira branch canal are shown in Table 1. By using the indirect measurement method, which is grain size analysis, hydraulic conductivity has been found. Afterwards, seepage losses are calculated using the Allen hazen empirical formula [4]. This value is obtained as 7.5 E-05 cum/m length (Table 3). The amount of water lost due to the seepage in Hajira branch canal in the hot season and all seasons has been shown in Tables 4 and 5, respectively. In the hot season, a total of 80 days were considered when the canal was in operation. From the calculation, the yearly seepage loss comes to around 15828480 m³/year. In terms of monetary value, considering the cost of 2.37 rupees/cumec, it is 3.75 crores. Similarly, the amount of water lost due to the seepage in all seasons comes around 43528320 m³/year, which is around 10.31 crores by considering 2.37 rupees/cumec. Table 6 shows the results of the computed average seepage losses for Hajira branch canal at different critical sections by the Davis and Wilson formula [5], Molesworth and yennidunia formula [6], Pakistani formula [7], Mortiz [8], Nazir Ahmad formula [9], and field results. The seepage losses by Davis and Wilson [5], Field test, Molesworth and yennidunia [6], Pakistani [7], Mortiz [8] and Nazir Ahmad formula [9] are 0.143694573 m³/s, 0.1144355 m³/s 0.04051233 m³/s, 0.017497216 m³/s, 0.008737758 m³/s, and 0.00872696 m³/s respectively. Moreover, comparative analysis has been done to find accurate results for the seepage loss. From the graph, it is visible that the Davis and Wilson and particle size methods gave applicable results when compared with the different actual measurements [10-12] (Figure 3 and Table 7).

Table 3: Determination of seepage losses by empirical formula.

Table 4: Seepage loss result.

Table 5: Seepage losses in canal in hot season.

Table 6: Seepage losses in canal in all season.

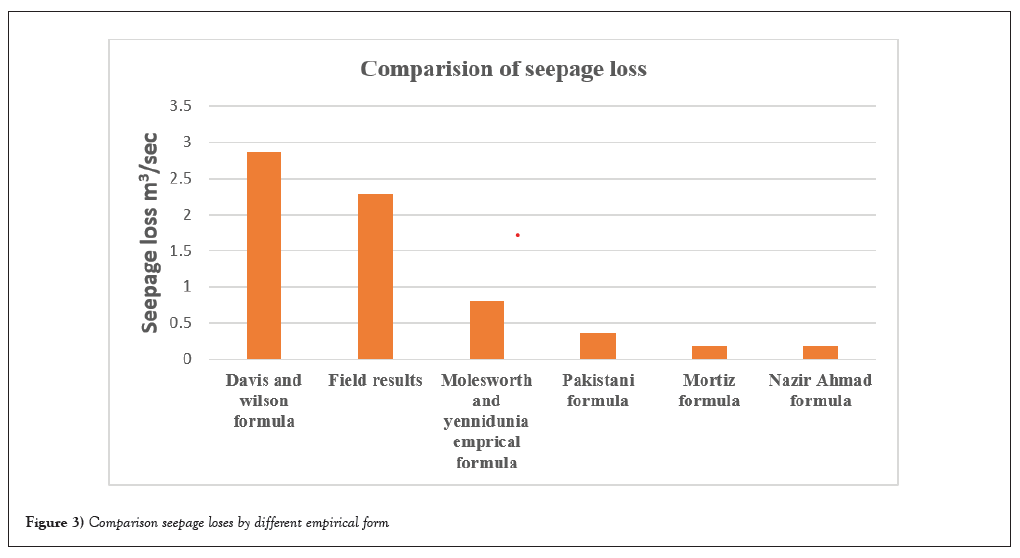
Figure 3: Comparison seepage loses by different empirical form.

Table 7: The results of the computed average seepage losses for Hazira canal at different critical sections by various formula.

Conclusion

Seepage losses of Hazira branch Canal were computed by empirical formulas at different sections along the total length of the canal. The study's conclusion emphasizes the need for a balanced approach, leveraging the convenience of empirical formulas while recognizing their limitations in capturing site-specific conditions accurately. Field tests remain pivotal in validating and refining these formulas, enhancing their applicability and ensuring more dependable estimations of seepage losses. The main conclusions can be summarized as follows:

1. The seepage losses are computed by using various empirical and particle size analysis method, from the results it is seen that Davis and Wilson Empirical and particle size analysis method gives good result.

2. We advise using Particle size method and Davis and Wilson Empirical Formula for computing seepage losses in Hazira branch canal.

3. Considering this much seepage losses in the canal, it is advised to provide canal lining in order to cover whole command area for irrigation.

References

1. Eshetu BD, Alamirew T. Estimation of seepage loss in irrigation canals of Tendaho sugar estate, Ethiopia. Irrig Drain Syst Eng. 2018; 7:3-7.

   [Crossref] [Google Scholar]

2. Uchdadiya KD, Patel JN. Seepage losses through unlined and lined canals. Int J Adv Appl Math Mech. 2014;2(2):88-91.

   [Google Scholar]

3. Ahuchaogu I, Etim I, Anwana E. Evaluation of seepage losses in an earth lined canal: a case study of University of Uyo Farm, Uyo, Nigeria. Int J Res Eng Sci Manag Res. 2015;2(2):2349-6193.

   [Google Scholar]

4. Sharma SK. Irrigation engineering and hydraulic structures. S Chand Publishing; 2017.

   [Google Scholar]

5. Akkuzu E. Usefulness of empirical equations in assessing canal losses through seepage in concrete-lined canal. J Irrig Drain Eng. 2012;138(5):455-460.

   [Crossref] [Google Scholar]

6. Hosseinzadeh Asl R, Salmasi F, Arvanaghi H. Numerical investigation on geometric configurations affecting seepage from unlined earthen channels and the comparison with field measurements. Eng Appl Comput Fluid Mech. 2020;14(1):236-253.

   [Crossref] [Google Scholar]

7. Khan AA, Hassan I, Muneer M. Evaluation of water losses in unlined canal: A case study of Malik Branch Canal, Bahawalnager, Pakistan. 2022.

   [Google Scholar]

8. Shah Z, Gabriel H, Haider S, et al. Analysis of seepage loss from concrete lined irrigation canals in Punjab, Pakistan. Irrig Drain. 2020;69(4):668-681.

   [Crossref] [Google Scholar]

9. Khan A, Khan TA, Sheraz K, et al. Relationship between seepage and discharge for Kabul River in district Nowshera. Pak J Agric Eng Vet Sci. 2015;31(2):249-259.

   [Google Scholar]

10. Arshad M, Zaman Q, Madani A, et al. Lining impact on water losses in watercourses: a case study in Indus Basin, Pakistan. In Annual Conference of the Canadian Society for Bioengineering. 2008;1-12.

    [Google Scholar]

11. Karad MM, Panke RA, Hangargekar PA. Seepage losses through canals and minors. Int J Eng Res Technol. 2013;2(11):1125-1134.

    [Google Scholar]

12. Syed NS, Shuqi Z. The use of empirical equations for seepage estimation in comparison with observed losses from irrigation canals. J Water Land Dev. 2023:21-29.

    [Google Scholar]